2023
Simons Collaboration
2 Simons Collaborations on Perfection in Algebra, Geometry, and Topology
48 mois – PI : Wiesława Nizioł
48 mois – PI : Pierre Colmez
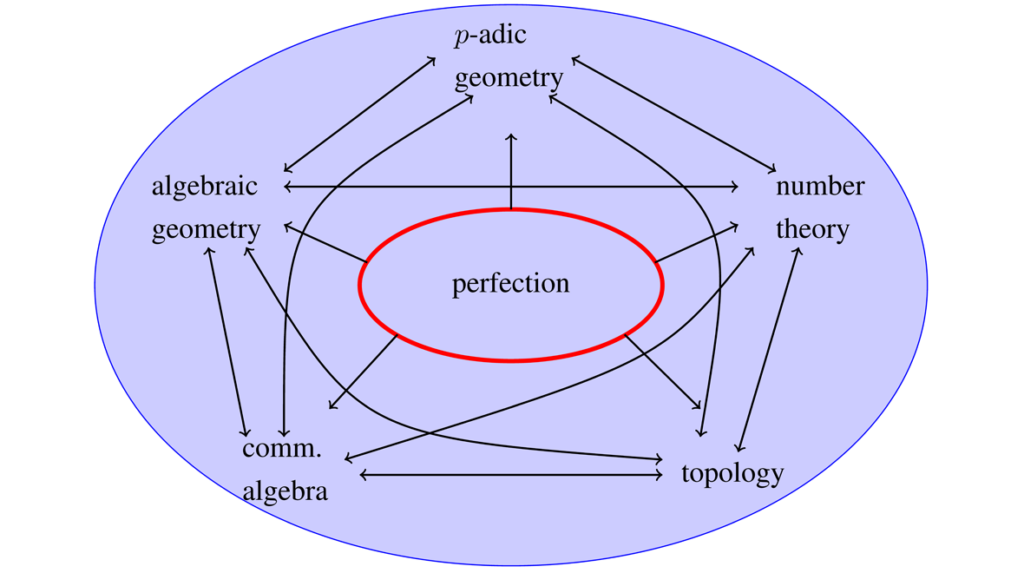
La fondation Simons finance une collaboration autour de la perfection en algèbre, géométrie et topologie, qui regroupe 13 membres dont Pierre Colmez et Wiesława Nizioł à Jussieu.
SIGMA – SinGular Monge-Ampère equations
Financement ERC CoG – 60 mois – PI : Eleonora Di Nezza
« Ce projet est motivé par la théorie M, la théorie des cordes en physique théorique et le Problème des Modèles Minimaux en géométrie algébrique. Nous étudions des espaces de Kähler singuliers en mettant l’accent sur leurs structures spéciales (d’origine géométrique différentielle) et leur interaction avec divers domaines de l’analyse.
Plus précisément, nous recherchons des métriques de Kähler singulières avec des propriétés de courbure spéciales, telles que les métriques Kähler-Einstein (KE) ou à courbure scalaire constante (cscK). Le problème de l’existence de ces métriques peut être reformulé en termes d’une équation de Monge-Ampère, qui est une équation aux dérivées partielles (EDP) non linéaire. Le cas KE a été résolu par Aubin, Yau (résolution de la conjecture de Calabi), et Chen-Donaldson-Sun (résolution de la conjecture de Yau-Tian-Donaldson) ; le cas cscK a été récemment résolu par Chen-Cheng (résolution d’une conjecture due à Tian). Cependant, ces résultats ne s’appliquent qu’aux variétés de Kähler lisses, et il est encore nécessaire de traiter avec des variétés singulières.
C’est là que la Théorie Pluripotentielle entre en jeu. Boucksom-Eyssidieux-GuedjZeriahi et l’auteur, avec Darvas et Lu, ont démontré que les méthodes pluripotentielles sont très flexibles et peuvent être adaptées pour travailler avec des équations de Monge-Ampère (singulières). Trouver une solution à ce type d’équations qui soit régulière en dehors du lieu singulier équivaut à l’existence de métriques KE ou cscK singulières.
À ce stade, un ingrédient crucial manque : la régularité de ces solutions (faibles). L’objectif principal de SiGMA est de relever ce défi. »
2021
MoCoS – Motivic Cohomology of Schemes
Financement ERC CoG – 60 mois – PI : Matthew Morrow
The project belongs to the field of arithmetic algebraic geometry and is centred around algebraic K-theory, motivic cohomology, and topological cyclic homology. The overall goal is to develop a general theory of motivic cohomology for arbitrary schemes, extending the existing theory of Bloch, Levine, Suslin, Voevodsky, and others in the special case of smooth algebraic varieties. This will describe non-connective algebraic K-theory via an Atiyah–Hirzebruch spectral sequence. The project relies on very recent breakthroughs in algebraic K-theory and topological cyclic homology In the case of singular algebraic varieties, our goal will be to develop a theory of motivic cohomology which both satisfies singular analogous of the Beilinson–Lichtenbaum conjectures and is also compatible with the trace maps to negative cyclic and topological cyclic homology. Its properties will refine those of K-theory in the presence of singularities; for example, we will study a motivic refinement of Weibel’s vanishing conjecture and a theory of « infinitesimal motivic cohomology » satisfying cdh descent. In the case of regular arithmetic schemes we will propose a new approach to the theory of p-adic motivic cohomology, based on topological cyclic homology and syntomic cohomology, which works in much greater generality than previous approaches. Perfectoid techniques will play an important role and we will establish the p-adic Beilinson–Lichtenbaum and Bloch–Kato conjectures.
2020
UnIntUniBd – Unlikely Intersection and Uniform Bounds for Points
Financement ERC StG – 60 mois – PI : Ziyang Gao
I propose to investigate the following long expected but widely open uniform bounds on rational and algebraic points. (1) Mazur’s conjecture on the number of points on curves, which implies the following two strong bounds : (1.i) the number of rational points on a smooth projective curve of genus g at least 2 defined over a number field of degree d is bounded above in terms of g, d and the Mordell- Weil rank ; (1.ii) the number of algebraic torsion points on a smooth projective curve of genus g at least 2 is bounded above only in terms of g. (2) Generalize the bound in (1) to higher dimensional subvarieties of abelian varieties. (3) Extend the bounds to semi-abelian varieties. Compared with existing results, the Faltings height is no longer involved in the bounds. The proofs I propose are via Diophantine estimates. Functional transcendence and unlikely intersections on mixed Shimura varieties play important roles in the proofs. Hence as pre-requests and extensions of the three goals listed above, I will also continue investigating on functional transcendence and unlikely intersection theories as well as their potential other interesting applications in Diophantine geometry. (lire la suite)
HyperK – Modern Aspects of Geometry : Categories, Cycles and Cohomology of Hyperkähler Varieties
Financement ERC SyG – 72 mois – PI : Olivier Debarre et Claire Voisin
Emanuele Macrì (Université Paris-Sud), Daniel Huybrechts (Bonn Universität, Allemagne), Claire Voisin (Collège de France-IMJ) et moi-même (Université de Paris-IMJ) avons obtenu une bourse ERC Synergy, qui débutera le 1er septembre 2020, pour une durée de 6 années. Le projet est intitulé HyperK et porte sur divers aspects de l’étude des variétés hyperkählériennes : catégories, cycles algébriques et cohomologie. Ces variétés complexes (aussi appelées variétés symplectiques irréductibles) constituent l’analogue en toute dimension paire des surfaces complexes dites K3, dont le programme d’étude, initié par A. Weil à la fin des années cinquante, est maintenant quasiment terminé. Notre ambition est de reproduire une version moderne de ce programme dans le cadre des variétés hyperkählériennes. Cela comprend l’étude de leurs catégories dérivées et des espaces de modules d’objets stables pour des conditions de stabilité de Bridgeland, celle de la filtration de Beilinson sur leurs groupes de Chow, et enfin la restriction des types cohomologiques, topologiques ou même des types de déformation possibles. (lire la suite)
2019
ROGW – Real and open Gromov-Witten theory
Financement ERC CoG – 60 mois – PI : Penka Georgieva
This proposal focuses on establishing new relations between natural objects in symplectic geometry with other fields of mathematics including knot and representation theory and the theory of integrable systems. All of these relations are motivated by theoretical physics. The main objects of study are moduli spaces of pseudo-holomorphic maps giving rise to real and open Gromov-Witten invariants. The classical Gromov-Witten invariants were introduced by Gromov at the birth of symplectic topology giving rise to obstructions to symplectic embeddings. Their interpretation by Witten as the coefficients of a partition function of a field theory placed them in a new light : striking dualities understood in physics relate them to mathematical objects of completely different nature and on completely different manifolds. This has and continues to generate enormous amount of high-level research aimed at understanding these relations better.(lire la suite) >> Voir le portrait vidéo de Penka sur la chaîne YouTube du CNRS.
EMERGENCE – Emergence of wild differentiable dynamical systems
Financement ERC CoG – 60 mois – PI : Pierre Berger
Many physical or biological systems display time-dependent states which can be mathematically modelled by a differentiable dynamical system. The state of the system consists of a finite number of variables, and the short time evolution is given by a differentiable equation or the iteration of a differentiable map. The evolution of a state is called an orbit of the system. The theory of dynamical systems studies the long time evolution of the orbits. (lire la suite)
HSD – Homeomorphisms in symplectic topology and dynamics
Financement ERC StG – 60 mois – PI :Sobhan Seyfaddini
The subject of this proposal is the field of continuous symplectic topology. This is an area of symplectic topology which defines and studies continuous analogues of smooth symplectic objects such as symplectic and Hamiltonian homeomorphisms and asks questions about persistence of various symplectic phenomena under uniform limits and perturbations.
Our aim is to explore, and further develop, continuous symplectic topology from two different perspectives : The first is a symplectic topological perspective which is informed by Gromov’s soft and hard view of symplectic topology. The second is motivated by the recent interactions of continuous symplectic topology and dynamical systems and it falls under the new field of symplectic dynamics. (lire la suite)
2018
SOS – Smooth Dynamics via Operators, with Singularities
Financement ERC AdG – 60 mois – PI : Viviane Baladi
The ergodic theory of smooth dynamical systems enjoying some form of hyperbolicity has undergone important progress since the beginning of the twenty first century, in part due to the development of a new technical tool : anisotropic Banach or Hilbert spaces, on which transfer operators have good spectral properties. Very recently, such tools have yielded exponential mixing for dispersing (Sinai) billiard flows (i.e. the 2D periodic Lorentz gas), which are the archetypal smooth systems with singularities. (lire la suite)
2017
ALMACRYPT – Algorithmic and Mathematical Cryptology
Financement ERC AdG – 48 mois – PI : Antoine Joux
Cryptology is a foundation of information security in the digital world. Today’s internet is protected by a form of cryptography based on complexity theoretic hardness assumptions. Ideally, they should be strong to ensure security and versatile to offer a wide range of functionalities and allow efficient implementations. However, these assumptions are largely untested and internet security could be built on sand. The main ambition of Almacrypt is to remedy this issue by challenging the assumptions through an advanced algorithmic analysis. (lire la suite)
GeoLocLang – Geometrization of the local Langlands correspondence
Financement ERC AdG – 60 mois – PI : Laurent Fargues
I formulated recently a conjecture that should allow to geometrize the local Langlands correspondence over a non-archimedean local field. This mixes p-adic Hodge theory, the geometric Langlands program and the classical local Langlands correspondence. This conjecture says that given a discrete local Langlands parameter of a reductive group over a local field of equal or unequal characteristic, one should be able to construct a perverse Hecke eigensheaf on the stack of G-bundles on the « curve » I defined and studied in my joint work with Fontaine. (lire la suite)



